JOURNAL PAPERS

 

Evaluation of elastic deformation energy in stone cutting of Portuguese marbles with a diamond saw

 

 

P. Falcao Neves; M. Costa e Silva; V.F. Navarro Torres

Cerena - 1ST, Lisbon, Portugal

 

 

---

SYNOPSIS

A detailed analysis of the geomechanical behaviour in rock cutting with a diamond saw is provided to obtain a better understanding of the mechanisms involved in the process. The energy of elastic deformation (before failure) and plastic deformation (post failure) was determined in uniaxial compression tests as well as the energy consumed per unit volume in the cutting process. The test samples are White and Ruivina marbles from Borba - Vila Vigosa in Portugal. Correlations between total deformation specific energy and cutting specific energy, normal and tangential forces and diamond indentation are analysed, and some conclusions are presented.

Keywords: cutting, specific energy, cutting sliding friction, indentation, diamond saw.

---

 

 

Introduction

Circular sawing with diamond-impregnated tools has been extensively used in stone processing plants, and prediction of rock cutting performance is important in the cost estimation and planning. Rock cutting or rock sawing performance depends on machine and diamond saw characteristics, depth of cut, sawing rate, tool wear, and rock properties.

The productivity and economics of the stone sawing operation are related to the mechanics of the cutting operation, as well as the wear resistance of the diamond tools. The relationships between forces, power, and energy have been extensively investigated for various types of stone machining processes, including sawing, cutting, grinding, and drilling^1-8.

A basic and fundamental parameter in stone cutting is the specific energy, which can be defined as the energy expended per unit of volume of material removed or per unit area of cut surface. The significance derives from the fact that any proposed machining mechanisms must be able to account for the magnitude of the specific energy and its dependence on the operating parameters. As a practical matter, the magnitude of the specific energy is especially useful for estimating the power requirements for a particular cutting operation. Unfortunately, the results reported by different researchers are difficult to compare due to the relatively narrow range of sawing or cutting parameters in each case and differences in the stone piece and diamond tools^6,7.

Several authors have proposed other methods to evaluate cutting and drilling performance using correlations between cutting specific energy, destruction specific energy and brittleness concepts^9-13.

Altindag Denkhausi4 suggest that brittleness is not a property of rock material but a term describing the type of fracture. Since fractures are seldom purely ductile or purely brittle, the scale of brittleness may be defined differently, depending on individual authors' suggestions^9-13, for example, by the ratio of elastic strain at fracture to plastic strain at fracture (B = e_Be Éå_Âñ). The higher this ratio, the higher the brittleness of the fracture and the lower its ductility. Brittleness may also be defined in terms of the ratio of specific elastic strain energy at fracture to total specific strain energy at fracture, by the ratio of the areas under the stress-strain curves (Figure 1).

 

 

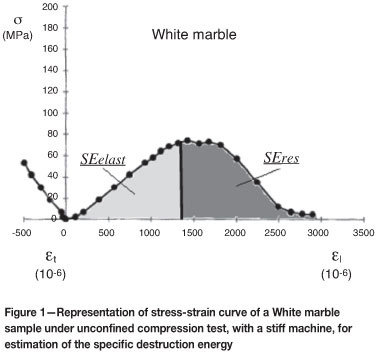
This work presents a methodology using a correlation between operating parameters (specific cutting energy) and a geomechanical parameter (total deformation specific energy) determined by current laboratory tests, using uniaxial compressive strength measured with a stiff machine. It seems to be a valid method for assessment of stone cutting performance with diamond saw, with the possibility of energy-saving control during the cutting process.

 

Rock cutting mechanics with a diamond saw

Nishimatsu15 presented a theory on the mechanics of rock cutting where he evaluates the cutting force based on the failure criterion of Mohr- Coulomb, the depth of cut, indentation and the geometry of cutting tool. The author concluded that, during the process of rock cutting, a wide variation of the cutting force and a discontinuous formation of cutting chips can be observed; there is no plastic deformation of cutting chips in rock cutting; these phenomena show that the mode of failure which takes place in rock cutting is a brittle one; the cutting force does not depend on the cutting speed. The author therefore, assumed that the cutting speed has no effect on the process and mechanics of rock cutting.

Nishimatsu¹⁵, observing the process of rock failure during cutting, and considering the similarity with the process of metal cutting, concludes that there is formation of a compacted crushed zone of material, which sticks to the edge of the diamond point or cutting tool, becoming part of this. The author also describes the initiation of a macroscopic failure crack, which leads to the formation of a coarse cutting chip, corresponding to a maximum value of cutting force applied. This cutting force induces a state of stress in the rock, causing the propagation of a macroscopic failure crack, when the normal and shear stress satisfy the criterion of rupture of Mohr-Coulomb.

The shape and size of rock chips produced during the cutting operation depend on the characteristics of the rock, the intensity of forces applied, and the technology used.

Experiments indicate that rock cutting is associated with a 'ductile' or a 'brittle' mode of failure depending on the depth of cut¹⁶. The ductile mode takes place at a shallow depth of cut and is associated with plastic flow, while the brittle mode occurs above a threshold depth of cut and is characterized by the propagation of tensile crack.

The mechanisms of wedge penetration, described in the literature, may help to clarify the phenomena, when a cutting tool (wedge of a diamond, for example) indents the rock.

Gnirk and Cheatham¹⁷ showed that the mechanism of indentation depends on the confining pressure. If such pressure is small, the behaviour is fragile, if it is large, behaviour tends to be ductile, and plastic deformation occurs (Figure 2).

 

 

In rock sawing with diamond disks, others authors¹⁸, describe the process with models based on simple geometries of cutting edges, and dividing the process of rock sawing into two mechanisms: primary chip formation and secondary chip formation (Figure 3). In this model, the process is influenced by several factors such as stone properties, forces between the stone and the diamonds points, state of stress distribution in the rock, and temperature in the rock-tool interface. The author also considers that the mechanical interaction between stone and tool is a result of forces caused by elastic and plastic rock deformation by cutting edges and friction between the stone and diamonds, stone and matrix, swarf (mix of rock fragments and coolant liquid) and matrix.

 

 

In front of the diamond wedge, the tangential force modifies the stress state in its neighbourhood. This zone is affected by tensile and compressive stresses. A zone of crushed stone and cracks appear. This mechanism is called primary chip formation^15,19.

While the rock shows an elastic characteristic up to its ultimate stress, it is necessary for cutting to reach a certain minimum cutting thickness. The material to be cut is deformed due to the compressive stress just below the diamond edge. When the load is removed, an elastic reversion leads to critical tensile stresses, which cause brittle fracture. This mechanism is termed secondary chip formation¹⁹. The swarf, or slurry, generated as a result of the process is carried away by the coolant.

 

Experimental procedures and analysis of the results

The analysis of the fundamental mechanics of disc cutting involves many complex factors such as characteristics of the saw (size, morphology, crystallographic properties and concentration of diamonds, hardness of the metallic matrix). It is clear that for a given pressure of the saw against the rock, the higher the concentration of diamonds in the matrix, the lower will be the force applied at each diamond edge. This should be closely related to the importance of the effects of several diamonds indentations in the stone, in the variation of the state of stress conditions, which certainly influences the mechanism of rock cutting.

To analyse this process, a set of cutting tests with diamond saw and uniaxial compressive tests were performed on two types of Portuguese ornamental marbles, White (Bi) and Ruivina (Ri), from the region of Vila Vigosa, Vigária. The results presented represent average values of marble samples from four different stone quarries covering an area of approximately 20 square kilometres and corresponding to 200 samples.

To make the results independent of operating conditions as much as possible it was decided to use a laboratory cutting saw machine with a down-cutting movement. Sharpening of the diamond saw was guaranteed by periodic cuts in blocks of cement mortar and sand. City water with constant flow was used as coolant liquid during all tests.

The cutting machine used in testing was a Diamant Boart, model AK 905 424 N (idling power Pw0 = 210 W and idling peripheral speed vp max = 21.6 mls) with a continuous rim saw blade (ds = 300 mm and b = 5 mm width, cutting point concentration of 30% and an active cutting point density (C) of 1É3 of the cutting point density). All workpieces were 20 mm thick (ap). For each cutting test, values were measured and calculated (according to the procedures used by Falcáo Neves3, and Xipeng Xu5,6) of the horizontal force (Fft), tangential force (F_t = PW/vp), feed rate (vf), and peripheral speed of the saw (v_p) during cutting (Figure 4). The net spindle power, Pw (w) was determined in each case by subtracting the idling power from total power. From the net spindle power, the specific energy SEcut, (Jlmm3), which is determined as the energy per unit volume of material removal, was calculated as4:

 

 

where Qw is specific removal rate per unit width (mm²/s) and b is the cutting width (mm) corresponding to the width of the saw blade.

The normal force component F_n can be calculated as⁷:

where F_h, F_v, and F_t are the horizontal, vertical, and tangential component forces applied as represented in Figure 4.

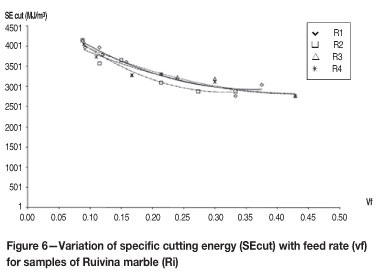
To evaluate the optimal conditions of the cutting operation for each sample (Figures 5 and 6), the minimum cutting specific energy (SE_mincut) was also estimated.

 

 

 

 

Uniaxial compressive tests were also conducted on the same types of ornamental marbles stones using a stiff compressive machine, Instron model 1340, with capacity of 4000 kN. Variations of the applied forces were recorded as well as the longitudinal strains before and after reaching failure. Total deformation (SE_totai), elastic (SE_dast), and residual (SE_res) specific energies were calculated according to the methodology suggested by Costa e Silva20.

The values presented in Table I are the averages for each type of test and for each sample.

 

 

Figure 7 shows correlations between the average values of minimum cutting-specific energy with total deformation-specific energy obtained from uniaxial compressive tests or the Portuguese marbles and for others types of rocks tested by Atici and Ersoyio. When the minimum specific cutting energy (SE_mincut) increases, the total deformation-specific energy (SE_total) also increases. The values obtained by Atici and Ersoyio show two clusters with very poor correlation, because those clusters represent two groups of rock types (limestones and granites) from different regions.

 

 

The correlations obtained with the Portuguese marbles (Figure 7) show that there is a good linear correlation between the minimum cutting specific energy and total deformation energy, and are in agreement with those obtained by Atici and Ersoy¹⁰. The objective of their work (Atici and Ersoyio) was the understanding of the mechanics during rock cutting, by using the correlation between total deformation-specific energy and minimum cutting-specific energy, measuring brittleness and relating it with optimum data obtained from experimental work.

The cutting-specific energy during sawing operations can be considered to be the consequence of several phenomena: chip formation (brittle fracture), plastic deformations, and friction components. As noted by Ertingshausen¹⁹, Tongshof¹⁸, and Xipeng^6,7 when testing granite samples, the fracture energy expended during the cutting operation may be considered to be negligible in comparison with the total energy consumed. Plastic deformation was also not obvious on the cutting surface in their experiments, so the energy should be expended mainly by friction sliding between the diamond saw edge and the rock surface.

Considering the influence of ap, depth of cut (mm) in the power consumption, Malkin4 defined the quantity A_a, mean chip longitudinal area (Aa = Lc.hm/V3), where the maximum undeformed chip thickness, h_m, (Figure 8) which is the maximum depth of cut taken by a cutting grain, can be expressed as4:

 

 

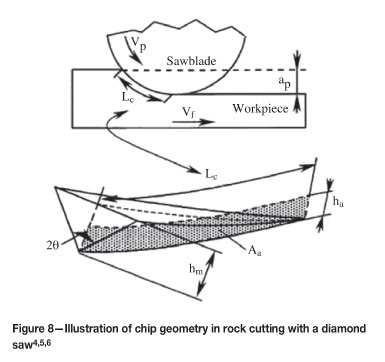
where è is the semi-angle for undeformed chip cross-section (for calculations è was considered to be 60°), C is the cutting point density (1/mm2), vf is the feed rate (m/s), v_p the peripheral velocity (m/s), and d_s the saw diameter (mm).

Plotting the power consumed per unit width (P'_W) versus overall rate of mean chip longitudinal areas generated per unit width (S_l, where Si is calculated by multiplying Aa by the number of cutting points per unit time per unit width of sawing), leads to the following expression4,5,6:

where λ is the ratio of L₁ to (L₁ + L₂) and Qw the specific removal rate (mm²ls).

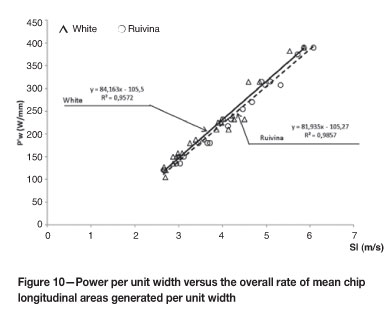
The energy component consumed due to the friction can be evaluated by the tangential force (F_t) versus normal force (F_n) and also by the power consumed per unit width (P'w) versus overall rate of mean chip longitudinal areas generated per unit width (Sl). Linear correlations (F_t = mFn + c) and (C'w = Js Sl + c') were obtained and are presented in Figures 9 and 10.

 

 

 

 

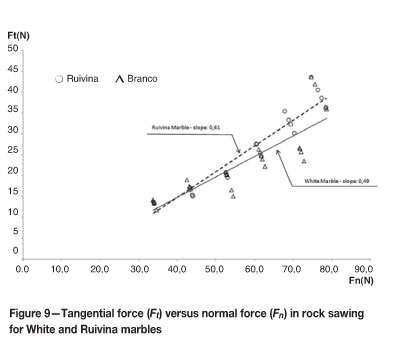
In Figure 9, the ratio between Ft and Fn (slope m) is constant for the ranges of feed rate for a given marble. As stated by Xipeng Xu&,7, the friction between the diamonds and the marble surface may be compared with the sliding friction law by Coulomb. Values of cutting friction coefficient are 0,49 for White and 0,61 for Ruivina Marble.

When comparing the cutting friction coefficient (m) values for both marbles (Figure 9), some hypothesis must be made. For the same value of normal force, the depth of indentation of the diamond edges along the depth of cut (a_p) for Ruivina marble is higher than for White marble. The of Ruivina chip fragments are larger than those of white marble, the coolant inflow remains constant (condition imposed in all tests), so the consequence is a decrease of the rate of removal of stone detritus, creating severe wear conditions due to higher friction between swarf, stone, and cutting tool.

The constant J_s (surface energy associated with sliding friction) is almost identical for both marbles, 84.16 KJlm2 for White marble and 81.94 KJlm2 for Ruivina marble (Figure 10). The small difference may indicate that an increment of energy is needed to indent the White marble, in comparison to Ruivina marble, for the same value of Sl because this parameter includes the mean chip thickness&,7 or mean diamond wedge indentation.

Analysing the variations (Figure 11) between forces (F_n and F_t) and maximum chip or mean chip thickness (h_max = ha for saws with continuous rim blade2i), the following conclusions can be drawn:

 

 

 Ruivina marble is easier to saw (to indent) than White marble

There is an increase of the mean chip thickness at a constant rate of application of the tangential force

 The tangential force does not interfere with the cutting mechanismis

 Accordingly to the literature and illustrated in this study, for high values of normal force applied to the rock surface, plastic deformation and friction may increase. This fact is verified with the decreasing rate of variation of normal force, Fn, for higher values of diamond indentation depths (h_max).

 

Conclusions

The state of stress imposed during the cutting action determines the rock brittleness behaviour during the cutting process. If the operating conditions are optimal, the plastic deformation and friction components of cutting-specific energy would be minimal. For high values of normal force applied there is an increase of cutting energy wasted in friction and plastic deformations.

Comparing the cutting friction coefficient (m) values for Ruivina and White marbles it was concluded that for the same value of normal force, Ruivina's depth of indentation (h_max) along the depth of cut (a_p) is higher than that for White marble. The Ruivina's chip fragments are larger than those of White marble. The coolant inflow remains constant (condition imposed in all tests), so the consequence is a decrease of the rate of removal of stone detritus, thus creating severe wear conditions due to higher friction between swarf, stone, and cutting tool.

The total deformation-specific energy value determined by current laboratory tests (uniaxial compressive strength with a stiff machine) seems to be a valid parameter for process assessment of stone cutting performance with diamond saw, because with the correlation obtained in this study it is possible to estimate the minimum value of specific cutting energy.

A good correlation (93%) was obtained between the total deformation-specific energy (SE_totaí) and the minimum cutting-specific energy (Se_mi_{n cut}) for the Portuguese marbles.

With this correlation it is possible to estimate the optimal conditions of operation of a cutting process when using a diamond saw, namely the most adequate feed rate (vf).

After establishing the optimal operational conditions for a particular type of Portuguese marble, the energy consumption calculation allows the estimation of overall operating costs in stone cutting with a diamond saw process.

It would be in the best interests for operators of stone cutting facilities to make use of correlations with common geomechanical parameters, in this case the uniaxial compressive strength, to evaluate optimal operating conditions on an industrial scale when using a diamond saw cutting process with different types of marbles or other ornamental stones.

 

References

1. KAHRAMAN, S. and GUNAYDIN, O. Indentation hardness test to estimate the sawability of carbonate rocks. Bulletin of Engineering Geology and the Environment, vol. 67, 2008. pp. 507-511.         [ Links ]

2. BRACH, K., PAI, D.M., RATTERMAN, E., and SHAW, M. C. Grinding forces and energy. Journal of Engineering/or Industry, vol. 110, 1988. pp. 25-31.         [ Links ]

3. FALCAO NEVES, P. Rock cutting geomechanics. MsSc Thesis. Technical Superior Institute, Technical university of Lisbon, 1993.         [ Links ]

4. MALKIN, S. Grinding Tecnology: Theory and Application of Machining with Abrasives. Wiley, New York, Reprint by SME, 1989.         [ Links ]

5. HWANG, T.W. Grinding energy and mechanism for ceramics. Ph.D. dissertation, university of Massachusetts, Amherst, uSA. 1997.         [ Links ]

6. XIPENG, X. FRICTION studies on the process in circular sawing of granites. Tribology Letters. J.C. Baltzer, (ed.) AG Science Publishers, vol. 7, 1999. p. 221-227.         [ Links ]

7. XIPENG, XU and LIAN, LI. Forces and energy in circular sawing and grinding of granite. Journal of Manufacturing Science and Engineering. Transactions of the ASME, vol. 123, 2001. pp. 13-22.         [ Links ]

8. COPUR, H., BILGIN, N., TUNCDEMIR, H., and BALCI, C. A set of indices based on indentation tests for assessment of rock cutting performance and rock properties. Journal of The South African Institute of Mining and Metallurgy, vol. 103, no. 9, 2003, pp. 589-600.         [ Links ]

9. ATICI, A. and ERSOY, A. Specific energy prediction for circular diamond saw in cutting different types of rocks using multivariable linear regression analysis. Journal of Mining Science, vol. 41, no. 3, 2005. pp. 240-260.         [ Links ]

10. ATICI, A. and ERSOY, A. Correlation of specific energy of cuttings saws and drilling bits with rock brittleness and destruction energy. Journal of Materials Processing Technology, vol. 209, 2009. pp. 2602-2612.         [ Links ]

11. GUNAYDIN, O., KAHRAMAN, S., and FENER, M. Sawability prediction of carbonate rocks from brittleness indexes. Journal of the South African Institute of Mining and Metallurgy, vol. 104, no. 4, 2004. pp. 239-244.         [ Links ]

12. GOKTAN, R.M. and YILMAZ, N.G. A new methodology for the analysis of the relationship between rock brittleness index and drag pick cutting efficiency. Journal of The South African Institute of Mining and Metallurgy, November, vol. 105, no. 10, 2005. pp. 727-733.         [ Links ]

13. ALTINDAG, R. Assessment of some brittleness indexes in rock-drilling efficiency. Rock Mechanics and Engineering, vol. 43, 2010. pp. 361-370.         [ Links ]

14. ALTINDAG, R and DENKHAUS, H.G. Brittleness and drillability: Comment on 'The evaluation of rock brittleness concept on rotary blast hole drills' and 'Correlation of specific energy with rock brittleness concepts on rock cutting'. Journal of The South African Institute of Mining and Metallurgy, vol. 103, no. 8, 2003. pp. 523-524.         [ Links ]

15. NISHIMATSU. THe mechanics of rock cutting. Journal of Rock Mechanics and Mining Science. Pergamon Press, vol. 9, 1972. pp. 261-270.         [ Links ]

16. DAGRAIN, F., RICHARD, T., and GERMAY, C. The Rock Strength Device : A scratching apparatus to determine rock properties. Proceedings of the 7th National Congress on Theoretical and Applied Mechanics (NCTAM2006), May 29-30, 2006. Faculté Polytechnique de Mons, Mons, Belgium.         [ Links ]

17. GNIRK, P.F. and CHEATHAM, J.B. An experimental study of single-bit tooth penetration into dry rock at confining pressures of 0-5000 psi. Transaction of the American Institute of Mining Engineers, no. 234 (II), 1965. pp. 117-130.         [ Links ]

18. TONSHOFF, H.K., HILLMANN-APMANN, H., and ASCHE, J. Diamond tools in stone and civil engineering: cutting principles, wear and applications, Diamond and Related Materials, vol. 11, 2002. pp. 736-741.         [ Links ]

19. ERTINGSHAUSEN, W. Wear processs in sawing hard stone. Industrial Diamond Review, vol 45, no. 5, 1985. pp. 254-258.         [ Links ]

20. COSTA e SILVA, M.M. Backfilling by tailings to the improvement of post-pillars behaviour, PhD Thesis, iST/uTL. 1995. Lisbon.         [ Links ]

21. KONSTANTY, J. Theoretical analysis of stone sawing with diamonds.Journal of Materials Processing Technology, Elsevier Science., vol. 123, 2002. pp. 146-154.         [ Links ]

 

 

Paper received Jun. 2011
Revised paper received Jan. 2012